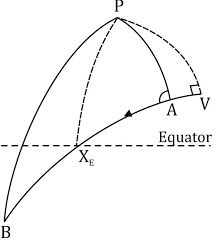
In Other words, we can understand the great circle as:
A great circle is the shortest path between two points along the surface of a sphere. A great circle is the intersection of the surface with a plane passing through the center of the planet.
The equator and all meridians are great circles. All great circles other than these do not have a constant azimuth, the spherical analog of slope; they cross successive meridians at different angles. The Gnomonic Projection represents arbitrary great circles as straight lines.
Great circles are examples of geodesics. A geodesic is the shortest possible path constrained to lie on a curved surface, independent of the choice of a coordinate system.